... estimator¹

It is a linear combination of the sample values.

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

... unbiasedness²

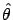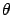An estimator $\hat{\theta}$ is an unbiased estimator of $\theta$ if the expected value of the estimator is the parameter to be estimate: $E[\hat{\theta}]=\theta$.

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

... consistency³

A consistent estimator is an estimator that converges in probability to the quantity being estimated as the sample size grows.

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

...#tex2html_wrap_inline5191#
⁴

Confidence Interval Lower Limit.

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

...#tex2html_wrap_inline5195#
⁵

Confidence Interval Upper Limit.

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

...#tex2html_wrap_inline5199#
⁶

Confidence Interval Mean.

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.