Complete closed form solution

The Complete solution improves upon the Simple solution in the sense that it is defined for all the input distributions 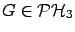${G}\in{\cal PH}_3$. Figure 2.13 shows an implementation of the Complete solution. Below, we elaborate on the Complete solution, and prove an upper bound on the number of phases used in the Complete solution.

Figure 2.13: An implementation of the Complete solution, defined for $G\in {\cal PH}_3$.