



Next: Simple closed form solution
Up: EC distribution
Previous: EC distribution
Contents
Theorem 3 implies that the parameter  of the
EC distribution can be fixed without excluding the distributions of
lowest variability from the set of EC distributions. In the rest of
the chapter, we constrain
of the
EC distribution can be fixed without excluding the distributions of
lowest variability from the set of EC distributions. In the rest of
the chapter, we constrain  as follows:
as follows:
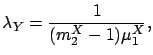 |
|
|
(2.3) |
and derive closed form representations of the remaining free
parameters ( ,
,  ,
,  ,
,  ,
,  ), where
these free parameters will determine
), where
these free parameters will determine 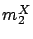 and
and 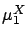 , which
in turn gives
, which
in turn gives  by (2.3). Obviously, at least three
degrees of freedom are necessary to match three moments. As we will
see, the additional degrees of freedom allow us to accept all input
distributions in
by (2.3). Obviously, at least three
degrees of freedom are necessary to match three moments. As we will
see, the additional degrees of freedom allow us to accept all input
distributions in  and to use a smaller number of phases.
and to use a smaller number of phases.
Takayuki Osogami
2005-07-19
![]() of the
EC distribution can be fixed without excluding the distributions of
lowest variability from the set of EC distributions. In the rest of
the chapter, we constrain
of the
EC distribution can be fixed without excluding the distributions of
lowest variability from the set of EC distributions. In the rest of
the chapter, we constrain ![]() as follows:
as follows: