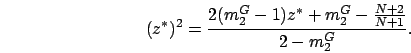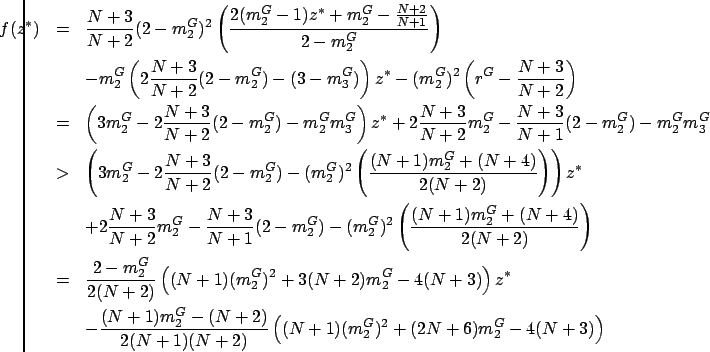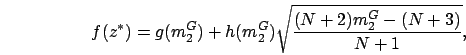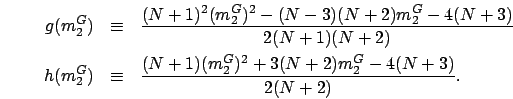Next: Proof of Theorem 8
Up: Proofs
Previous: Proof of Lemma 5
Contents
For each case, it is easy to check, by substitution, that conditions
(2.14)-(2.16) are satisfied.
Below, we prove condition (2.13) and 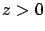 .
.
We begin with the first case, where 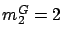 .
It is easy to see (2.13) is true
if
.
It is easy to see (2.13) is true
if 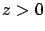 , since
, since
Further, 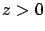 if
if
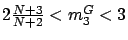 ,
which is true by
,
which is true by
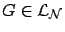 ,
,
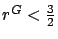 , and
, and 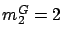 .
.
Below, we consider the second case, where 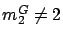 .
We first prove
.
We first prove 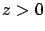 by showing that
by showing that  is the
larger solution of the two solutions of a quadratic equation
that has a unique positive solution.
Observe that
is the
larger solution of the two solutions of a quadratic equation
that has a unique positive solution.
Observe that
Thus,  is a solution of the following quadratic equation:
is a solution of the following quadratic equation: 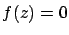 , where
, where
Since the coefficient of the leading term,
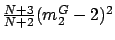 ,
is positive and
,
is positive and 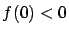 ,
there exists a unique positive solution of
,
there exists a unique positive solution of 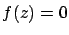 .
.
Second, we show
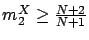 . We consider two cases:
(i)
. We consider two cases:
(i) 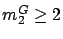 and (ii)
and (ii) 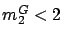 .
Case (i) is easy to show. Suppose
.
Case (i) is easy to show. Suppose 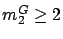 .
Observe that by (2.15),
.
Observe that by (2.15),
Thus, if 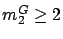 , then
, then
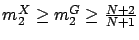 .
Below, we consider case (ii).
.
Below, we consider case (ii).
Suppose 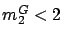 .
Observe that
.
Observe that
again by (2.15).
Thus,
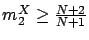 iff
iff
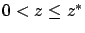 , where
, where 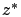 is a larger solution,
is a larger solution, 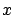 , of the following quadratic equation:
, of the following quadratic equation: 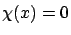 , where
, where
That is,
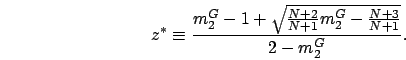 |
(A.1) |
Thus, it suffices to show 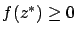 .
Since
.
Since 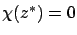 ,
we obtain
,
we obtain 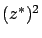 as a linear function of
as a linear function of 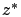 :
:
By substituting this 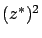 into the expression for
into the expression for 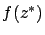 , we obtain
, we obtain
where the inequality follows from the assumption on 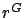 in the lemma.
By substituting (A.1)
into the last expression, we obtain
in the lemma.
By substituting (A.1)
into the last expression, we obtain
where
Since
for
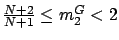 ,
,
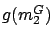 and
and 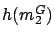 are increasing functions of
are increasing functions of
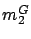 in the range of
in the range of
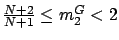 .
Since
.
Since
we have
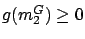 and
and
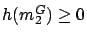 for
for
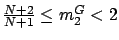 .
This implies
.
This implies 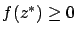 .
width 1ex height 1ex depth 0pt
.
width 1ex height 1ex depth 0pt




Next: Proof of Theorem 8
Up: Proofs
Previous: Proof of Lemma 5
Contents
Takayuki Osogami
2005-07-19
![]() .
It is easy to see (2.13) is true
if
.
It is easy to see (2.13) is true
if ![]() , since
, since
![]() .
We first prove
.
We first prove ![]() by showing that
by showing that ![]() is the
larger solution of the two solutions of a quadratic equation
that has a unique positive solution.
Observe that
is the
larger solution of the two solutions of a quadratic equation
that has a unique positive solution.
Observe that
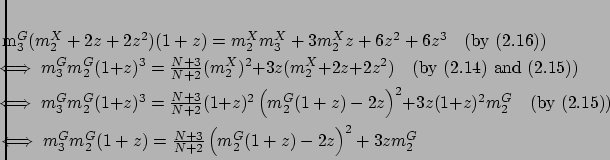
![]() . We consider two cases:
(i)
. We consider two cases:
(i) ![]() and (ii)
and (ii) ![]() .
Case (i) is easy to show. Suppose
.
Case (i) is easy to show. Suppose ![]() .
Observe that by (2.15),
.
Observe that by (2.15),
![]() .
Observe that
.
Observe that